
In particular, it must also be true for specific values of. This equation can be considered two functions of which are equal to each other for all values of. Is NOT GENERAL ENOUGH and will not always lead to a correct partial fractions decomposition. Since is an irreducible quadratic expression, assuming only that ěegin by factoring the denominator, getting Find a partial fractions decomposition for

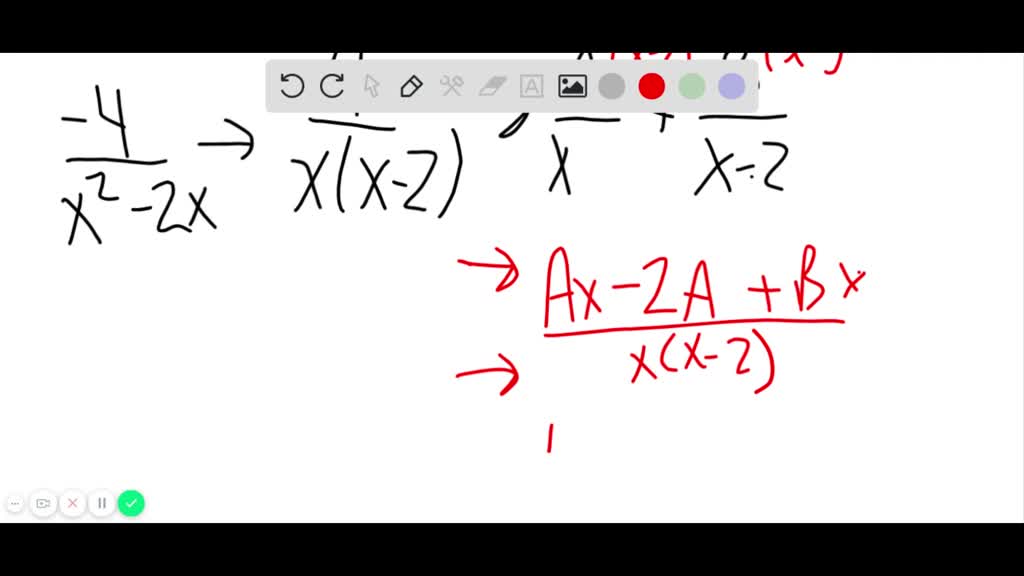
In addition, if two complex numbers are equal, then their real and complex components are equal. Where the rational function on the right-hand side of the equation satisfies condition 2. For example, polynomial division leads to If the degree (highest power) of is equal to or greater than the degree of, then you must use polynomial division in order to rewrite the given rational function as the sum of a polynomial and a new rational function satisfying condition 2 above. Here is another important point to consider when applying the method of partial fractions to the rational function After getting familiar with this process, in order to save some time, get in the habit of going from the step at equation (*) directly to the step at equation (**). However, any other two choices for will lead to the exact same values for and (after solving two equations with two unknowns). It should be noted that and were chosen for use in equation (**) for their convenience of ``zeroing out" terms in the equation. We can now say that a partial fractions decomposition for The right-hand side of this equation can be considered a function of which is equal to 6 for all values of. Since the fractions in the above equation have the same denominators, it follows that their numerators must be equal. (Get a common denominator and add the fractions.) the degree (highest power of ) of is smaller than the degree of. both and are polynomials (constants together with positive integer powers of only) It can be shown that such constants always exist for the rational function Now ASSUME that there are constants and so that ? Begin by factoring the denominator, getting For example, what would be a partial fractions decomposition for Of course, what we would like to be able to do is find a partial fractions decomposition for a given function. This concept can also be used with functions of. So that we can now say that a partial fractions decomposition for This method is based on the simple concept of adding fractions by getting a common denominator. THE METHOD OF INTEGRATION BY PARTIAL FRACTIONSĪll of the following problems use the method of integration by partial fractions. You may switch on the 'Show details' toggle of the calculators above to study the procedure steps using an example.Integration by Partial Fractions Next: About this document.
equate each coefficient of P 2(x) to the linear expression with a jk, b jk,c jk corresponding to the same degree of x.expand the numerator polynomial factors and express the numerator polynomial coefficients in terms of linear expression of unknown constants a jk, b jk,c jk.reduce the right side numerator to a common denominator., where a jk, b jk,c jk are real numbers. then the partial fraction decomposition takes the form:.find the denominator factorization as l linear factors for real roots of Q 1(x) and n quadratic factors for complex roots of Q 1(x):.if the degree of P 1(x) is greater than or equal to the degree of Q 1(x), do the long division to find the common polynomial term (quotient) and the new numerator P 2(x) (remainder), which degree is less than Q 1(x) degree:.

convert the denominator polynomial to monic by dividing P (x) and Q (x) by the leading coefficient of Q (x).The partial fraction decomposition procedure of a polynomial fraction P(x)/Q(x) is as follows:


 0 kommentar(er)
0 kommentar(er)
